Does the Altitude of an Equilateral Triangle Bisect the Base

In every other triangle the altitude does not bisect the base. This line containing the opposite side is called the extended base of the altitude.

How To Find The Height Of An Equilateral Triangle Intermediate Geometry

ADC and CDB are equal because the have two sides equal and one angle equal SAS then ADDB hence the altitude bisects the base.

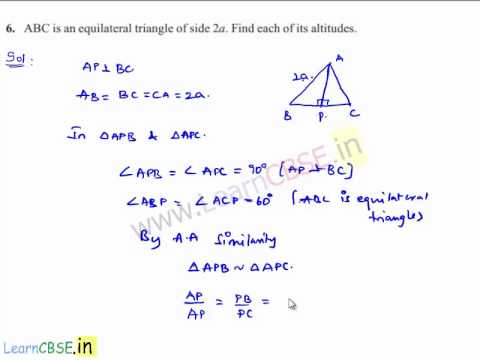
. In an equilateral triangle a triangle with three equal sides an altitude cuts any side perfectly in half meaning this is now called the altitude bisector. In geometry an altitude of a triangle is a line segment through a vertex and perpendicular to ie forming a right angle with a line containing the base the side opposite the vertex. An obelisk is a tall thin four sided monument that tapers to a pyramidal top.

Also in the ET and IT where the. Does the Altitude of a Triangle Always Make 90 With the Base of the Triangle. That depends how perpendicular is drawn if perpendicular is drawn from opposite vertex it will be an Altitude and if it bisects the base of.

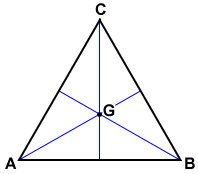
From what I deduced from Wikipedia is that this is only true if the triangle is either isosceles or a right triangle is not fully correct. The three altitudes extending from the vertices A B and C of ABC above intersect at point G. That way you will not forget them.

When the altitude to the base of an isosceles triangle is drawn two congruent triangles are formed proven by Hypotenuse - Leg. Yes by definition it has to be. Show that the bisector of the vertical of.

Then that will imply that the angles at the base of the altitude are also equal so they are both 90 degrees. Since the altitudes are the angle bisectors medians and perpendicular bisectors point. Only in an equilateral ET and isosceles triangle IT would that be true and in the isosceles the non-equal side would have to be the base.

The three altitudes of an equilateral triangle intersect at a single point. Figure 2 In a right triangle each leg can serve as an altitude. An altitude from a vertex bisects the opposite base if and only if the two sides emerging from that particular vertex are equalnot necessary in a right angle triangleTherefore you need to specify this condition before assuming that the altitude cuts.

Now if we drop an altitude from the apex of the triangle to the base it divides the triangle into two equal right triangles. And so on III. This means the angle bisector is also the height to the base.

_____ 51 Isosceles Equilateral Triangles An altitude is a perpendicular segment from a vertex to the line containing the opposite side. We use congruent triangles to show. Yes the altitude of a triangle is a perpendicular line segment drawn from a vertex of a triangle.

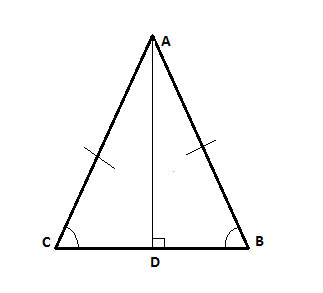
With these two facts in hand it will be easy to show several other properties of isosceles triangles using the same method triangle congruency. In Figure 3 AM is the altitude to base BC. Equilateral triangles have only one shape or design.

In Figure 2 AC is an altitude to base BC and BC is an altitude to base AC. Use the law of sines to show that the base is divided into equal lengths. As we know an equilateral triangle has all equal sides.

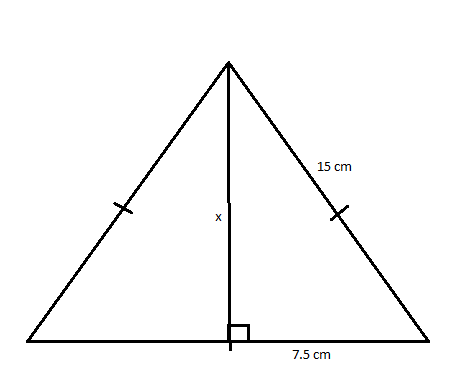
Here we will show the opposite. That the angle bisector is perpendicular to the base in an isosceles triangle. Thus from the above figure we can find the height h of the equilateral triangle as.

It is interesting to note that the altitude of an equilateral triangle bisects its base and the opposite angle. CD is the altitude from vertex C to base AB. It bisects the base of the triangle and always lies inside the triangle.

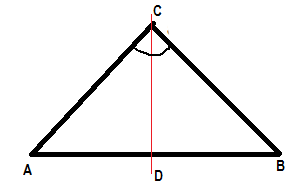
I interpret your question asking would it bisect if a dotted line. Hence this median is also the altitude. The altitude to the base of an isosceles triangle bisects the base.

An altitude of an equilateral triangle is also an angle bisector median and perpendicular bisector. If median drawn from vertex A is also the angle bisector the triangle is isosceles such that AB AC and BC is the base. Try to prove all these properties on your own.

Having proven the Base Angles Theorem for isosceles triangles using triangle congruency we know that in an isosceles triangle the legs are equal and the base angles are congruent. Does the altitude of a triangle bisect the angle it starts from. Altitudes of equilateral triangles.

The altitude to the base of an isosceles triangle bisects the base. In this proof and in all similar problems related to the properties of an isosceles triangle we employ the same basic strategy. Figure 3 An altitude for an obtuse.

Altitudes can sometimes coincide with a side of the triangle or can sometimes meet an extended base outside the triangle. It is also called altitude of an equilateral triangle. The altitude to the base of an isosceles triangle bisects the vertex angle.

The image below shows an equilateral triangle ABC where BD is the height h AB BC AC ABD CBD and AD CD. Lets start by proving that in. The altitude or height of an equilateral triangle is the line segment from a vertex that is perpendicular to the opposite side.

A few things this. Answer 1 of 2. In an equilateral triangle each altitude median and angle bisector drawn from the same vertex overlap.

The intersection of the extended base and the altitude is called the foot of the altitude.

Height To Base Of An Isosceles Triangle Bisects The Base Geometry Help